Find the Kirillian!--the Sandragon puzzle seems a bit arbitrary.

The Galactic Pirate--the tunnels puzzle(follow Marko Khen down the tunnel and run into a Zapf if you go the wrong way) is arbitrary, unless I am missing something.

Robot World--this is in sector 33 although The Galactic Pirate mentions your next business is in sector 82.

Space Olympics--

Monsters of Doorna--

Star Crystal--the puzzle about traversing a mobius strip is, technically, WRONG, given the picture on page 40, but it is correct if you make a copy of the Mobius express(_do_ photocopy pages 119 and 120 and cut the copies out!)
But to spare those of you without a photocopier the trauma of cutting the Mobius Express out of The Star Crystal, I offer a visual explanation below. You can follow along if you take a narrow strip of paper and color it as I've shown below.

Front of mobius strip	Back of mobius strip

Perhaps less relevant but interesting as well is the case of the changing spy badge. On page 12 we are not sure of the number of sides on the badge. However, note that if we are seeing the full badge(i.e. it is not cut off anywhere) then it has at eight points on page 12. If either the top or the bottom is cut off but not both, the badge has seven points. If it is cut off on both the top and bottom, the badge has six points. But then the badge on page 12 is not the same as the badge on page 22. Note that on page 22, the top and bottom of the badge are points of a hexagon. On page 12, they are lines of a hexagon. To put it another way, the spy's image on the badge has been rotated thirty degrees!

<--p. 12 on left, p. 22 on right-->

Rebel Spy--

Mission to Microworld--

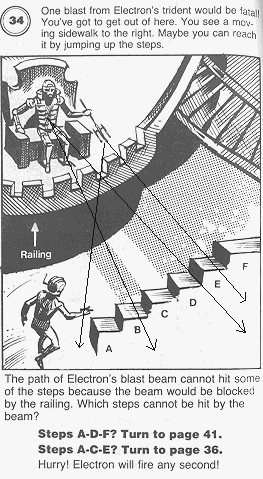First, the puzzle with Electron's trident is annoying. The main problem is that you don't know whether the ridges in the castle wall should block you from Electron or his trident. Here is a diagram where I drew in four arrows, scanned directly from the book. Electron can see over the railing, but he can't point his trident at the same squares. You get zapped on page 41 (for touching step D, I assume) but this diagram seems to indicate that the writers got this puzzle wrong.

Here is a very interesting "over/under" puzzle which seems to be one of the most thought-provoking puzzles in the series. I transcribed the basic layout from page 24. You can fly over solid barries and under clear ones. However, you can't fly over or under two in a row, or you will be killed. You start on the bottom, having crossed the middle solid barrier. You must cross alternately through solid and non-solid lines to move between grid squares. How do you get "out"(to the top?) There are two choices the book gives you--the second from left or second from right. Well, it turns out you don't need to get through this maze. There's a way to tell without going through this maze and another way to tell by going through a very different looking maze. The first solution notes this point: Whether you can pass through solid or clear barriers depends only on which cell you're in. Why?

Brief proof: Let's say you moved x squares left and y squares up from your starting point. x and y needn't be positive. Then let x1=your moves up, x2=your moves down, y1=moves left, and y2=moves right. Then Total moves=x1+x2+y1+y2=(x1-x2)+(y1-y2)=x+y-2(x2)-2(y2). In other words, # of total moves(which determines which barrier you can cross)=total #of squares you are away from origin + (some even #).
So if x+y is odd, your number of moves is odd, and you must go through a solid barrier. If x+y is even, your number of moves is even, and you must go through a clear barrier.
Now point A(the second from left square) is 7 units up and 3 left of the start, for 10 units total. You can go through a clear barrier, and since there is one on the upper border, you may be able to exit from point A.
However, point B(the second from right) is 10 units away, too, but it has a solid barrier to the north. That is not a legal move, and maybe your last few moves were okay, but somewhere along the line you violated the pattern.
It turns out you can exit through all upper barriers except the 1st, 3rd, and 4th from the left. How? Because each barrier can only be crossed from one direction. Pick a vertical barrier. If it can be crossed from the square to its left, then consider the moves it took to get to the square to the left and right. One is odd, and one is even. So the square on the right cannot cross through the same barrier as the one on the left. However, each square must be able to cross through a barrier. Our choice was arbitrary so this proof works for horizontal barriers, etc. This means that the maze is a conglomerate of one-way doors. Here's what they look like(screen shot cut and pasted from WordPad):

Ultraheroes--One puzzle here appears to be type-set wrong. On page 81, the rooms seem to have "east" and "west" flipped. The path to the left(east) is clearly shorter but you hit another puzzle when you go that way. If "east" and "west" were flipped, then north would be up(which is sort of the default for maps) and the puzzle would be OK.

Oh yeah. In The Star Crystal you see that Tunk doesn't speak English. Either your Interplanetary Spy stopped off in a Douglas Adams book and got himself a Babel Fish or Tunk learned. And besides that, the geyser-style hair is a lot bigger in Ultraheroes and in fact Kort in Ultraheroes looks more like Tunk in The Star Crystal than Tunk in Ultraheroes.

Planet Hunters--one puzzle here seems to be bad. The cube puzzle with Venya is done the wrong way.

Red Rocket--the most heinous error here is the puzzle with Sarvala and Venya and Gradak. You start out with pieces in the following order:

Sarvala

Venya

Gradak

[Open]

You can jump one figurine over another or just move a figurine a square, and you wish to reach:

[Open]

Gradak

Venya

Sarvala

When you solve the puzzle, Sarvala lifts up the blank square next to him. Shouln't that be where Venya is? Seems like Gradak is the only one next to an open square!

This puzzle is interesting for other reasons, though--you must take an even number of steps to solve it. Why? Consider this: don't worry about how large the board is at first or about two figurines being on top of each other. Note that you have to move Gradak one square, Venya one square, and Sarvala three squares. But you must switch Venya and Gradak, Sarvala and Gradak, and Venya and Sarvala in some order. With each switch, a figurine moves two squares. So after the three switches, the figurines have to move an odd number of squares. That requires an odd number of moves(see the argument above--if they need to move x squares, let's say they move y to the left and y+x to the right. That's a total of 2y+x which is even iff(if and only if) x is. So 3 switches+an odd number of non-jumps=an even number of moves.

This puzzle cannot be done in less than six moves. Why? Note that there must be three jumps. Let Sarvala, Gradak, and Venya start on squares 1, 2, 3, and 4 respectively. They wind up on 4, 3, and 2. Now a jump moves you from an odd number to an odd number, or an even to an even(mathematically speaking, it "preserves the parity.") So at the beginning and after three jumps, Gradak, Venya and Sarvala are on squares of wrong parity. Each must move at least once to restore the parity, meaning you need at least six moves. But since you need an odd number of one-square shifts, the number of moves to complete the puzzle is even. They give you a choice between 5 and 6 but adding 8 in would be very clever.
This puzzle actually has two solutions: 3-4, 1-3, 2-1, 4-2, 3-4, 1-3. Also it has 2-4, 1-2, 3-1, 4-3, 2-4, 1-2. These moves are forced if you want to complete the puzzle in six moves.

Skystalker--the very first puzzle asks you to look for a circle half as big as one given. The other two circles(1 and 2) have radii that are about .7 and .5 times the radius of the original, respectively. Since we are wondering how big they are, not about how long they are, we remember that area varies as the square of the radius. Therefore circle 1 is about .49 as big as the original, and circle 2 is .25 as big. That you aren't killed if you choose circle 1 saves the author's(or more likely an editor's who "never really did understand that math stuff" and, well, edited) bacon a bit, but this puzzle is probably the most blatantly wrong of them all.

Back to the main page.

Any feedback? Mail me!